
Visualizing the E8 root system
The E8 root system consists of 240 vectors in an eight-dimensional space. See what is E8? Those vectors are the vertices (corners) of an eight-dimensional object called the Gosset polytope 421. In the 1960s, Peter McMullen drew (by hand) a 2-dimensional representation of the Gosset polytope 421. The image shown below was computer-generated by John Stembridge, based on McMullen's drawing.The lines in the picture connect adjacent vertices in the polytope, with colors chosen according to the length of the 2-dimensional projection. Since the picture is a 2-dimensional projection of an 8-dimensional object, it captures only some of the symmetries of the Gossett polytope.
We thank John H. Conway, mathematics professor at Princeton University, for pointing out to us the connection between E8 and the Gosset polytope 421.
The Lie algebra E8 is 248-dimensional: the 8-dimensional space depicted here, plus one dimension for each of the 240 root vectors.
Picture also available as a high resolution EPS file or PDF file.
A scan of a copy of Peter McMullen's drawing available as a 300 dpi PDF (2 Meg). Please contact AIM if you know the location of McMullen's original drawing of the Gosset polytope 421.
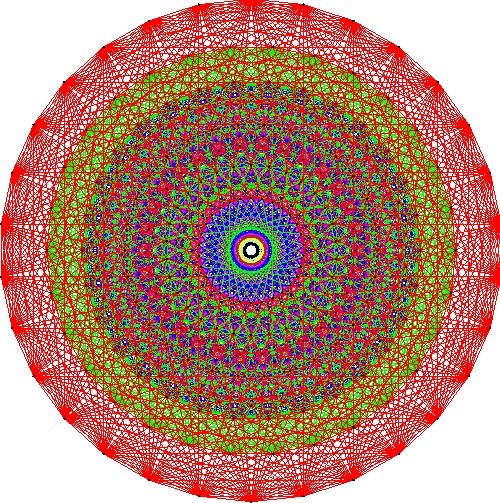
For more details about Coxeter planes and pictures of root systems, see John Stembridge's web page.
More information about E8 and the Gossett polytope, and a 3-dimensional Zome model, can be found on David Richter's website.