Analytic number theory

The motivation for studying the zeros of the zeta-function is the precise relationship between the zeros of $\zeta(s)$ and the errot term in the prime number theorem. Define the Von Mangoldt function by

$$\Lambda(n)=\begin{cases}\log p &\hbox{ if $n=p^k$ }\cr 0 & otherwise, \cr \end{cases}$$

where $p$ is a prime number. Then

$$\begin{eqnarray*} \psi(X):=&& \sum_{n\le X} \Lambda(n) \cr =&& Li(X) + O(X^{\sigma_0+\varepsilon}) \end{eqnarray*}$$

if and only if $\zeta(s)$ does not vanish for $\sigma>\sigma_0$. There $Li(X)$ is the logarithmic integral

$$Li(X)=\int_2^X \frac{dt}{\log t} .$$

In particular, the best possible error term in the prime number theorem is $O(X^{\frac12 + \varepsilon})$, which is equivalent to the Riemann Hypothesis.

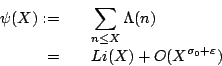
Similarly, the Generalized Riemann Hypothesis is equivalent to the best possible error term for the counting function of primes in arithmetic progressions.

Riemann Hypotheses for other $L$-functions have not yet been shown to be strongly connected to the distribution of the prime numbers.

Back to the main index for The Riemann Hypothesis.