Fundamental domains
and zeros of cusp forms

Dots in a vertical strip with an open top and curved bottom.
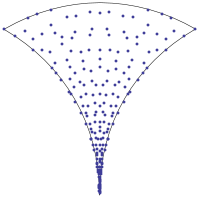
This picture shows the zeros of a weight 2000 Hecke eigenform in a particular fundamental domain for SL(2,Z). The image on the main QUE page shows the zeros of a weight 500 cusp form. Weight k forms have k/12+O(1) zeros.    
Dots in a triangle with curved sides.
Image courtesy of Fredrik Stromberg

Zeev Rudnick proved that QUE implies that the zeros of the associated cusp forms also are equidistributed in the (hyperbolic) upper half-plane. So this picture is an illustration of the result of Holowinsky and Soundararajan.

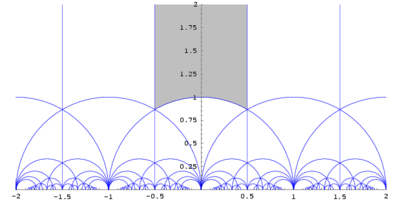
The standard fundamental domain for SL(2,Z) is the set of points

D= { z=x+i y : -1/2 < x < 1/2   and   |z|>1 }

in the upper half-plane. This is the shaded region in the picture below. The picture above shows a different (but equivalent) fundamental domain. It was chosen because in the usual fundamental domain the points near the cusp at infinity are very far from the bulk of the points (in the Euclidean metric). But if you would like to see the above picture transformed into the standard fundamental domain, just scan down the left edge of this webpage.

The upper half-plane tiled by fundamental domains for SL(2,Z):