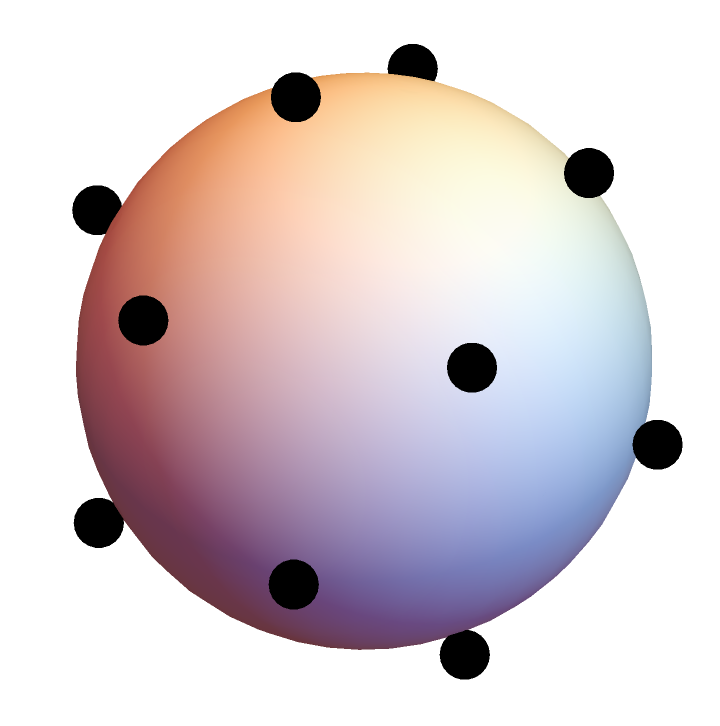
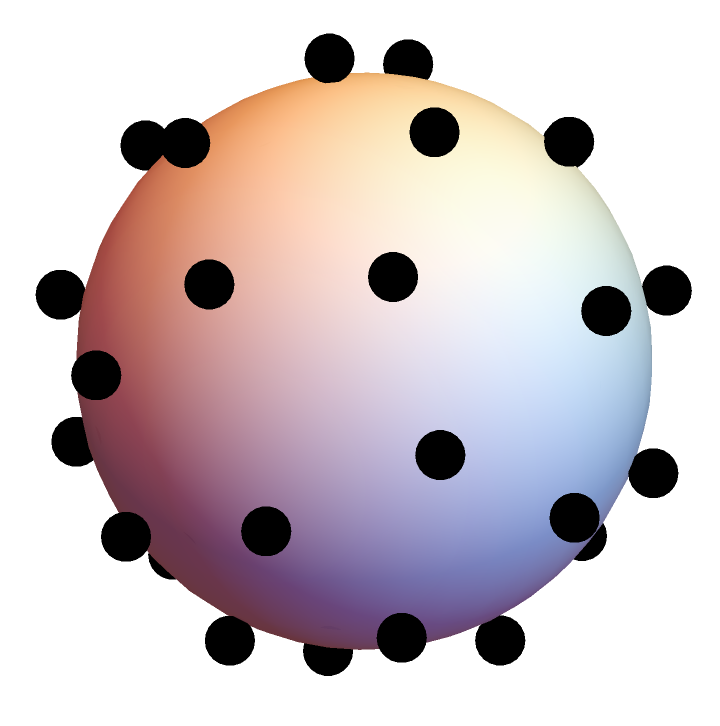
The recipients of the 2024 Alexanderson Award are Dmitriy Bilyk, Alexey Glazyrin, Ryan Matzke, Josiah Park, and Oleksandr Vlasiuk, for their paper “Energy on spheres and discreteness of minimizing measures,” published in the Journal of Functional Analysis in 2021.
The Alexanderson Award is given annually to a notable paper arising from an AIM activity, which has been published in the past 3 years. This paper includes work arising from the 2018 AIM workshop “Discrete geometry and automorphic forms.”
The Alexanderson Award committee, made up of members of the AIM Scientific Board, selected this well-written paper from the elegible papers during the review period based on its universal appeal, the centrality of the problem, and its impact on the field in the short three years since publication.
This work concerns equidistribution on spheres. The simplest version of the problem is: how can you distribute $n$ points on a basketball as uniformly as possible? A moment’s thought suggests that for most $n$, the answer is not pretty. The answer also depends on the specific definition of “uniformly.” Is the goal to have the points as far as possible from each other? Or to have every location on the sphere close to one of the given points? An approach which generalizes to the topic of the winning paper is to give each point an identical electric charge and then minimize the total energy.
Energy minimization on the sphere arises naturally in many contexts, including mathematical physics, discrete and metric geometry, coding theory, and signal processing. This article focuses on “attractive-repulsive potentials” on the sphere. These are functions which are increasing near $1$ but decreasing near $−1$; in other words, two particles experience repulsion when they are close and attraction when they are far apart.
If $f:[-1,1]\to \mathbb R$ is measurable and bounded below, then the continuous energy integral on the $d$-dimensional sphere $\mathbb S^d$ is defined for any Borel probability measure $\mu$ as
$$
I_f(\mu) = \int\limits_{\mathbb S^d} \int\limits_{\mathbb S^d} f(\langle x, y\rangle) d\mu(x) d\mu(y) .
$$
Given the function $f$, the goal is to find a measure $\mu$ for which $I_f(\mu)$ is minimized. Note that such a measure typically is not unique: the sphere has a lot of symmetry, so any symmetry of the sphere will move one minimizing measure to another minimizing measure.
This paper focuses on one of the simplest and most natural attractive-repulsive potentials, given by $f(t) = |t|^p$. The energy in this case is called the “$p$-frame energy.” The easiest case is when $p$ is an even integer. It turns out that in that case the constant measure is a minimizer.
The surprising conjecture is that in every other case, any minimizing measure is discrete. The authors make significant progress on that conjecture, showing that if $p$ is not an even integer then the support of any minimizing measure for the $p$-frame energy has empty interior.
For a variety of other functions $f$, the authors prove the full conjecture that there exists a discrete minimizing measure, and furthermore they find a bound on the number of points in the support. Examples of such $f$ include certain functions related to the Gegenbauer polynomials.
